Wheel and axle

The wheel and axle is one of six simple machines identified by Renaissance scientists drawing from Greek texts on technology.[1] The wheel and axle consists of a wheel attached to a smaller axle so that these two parts rotate together in which a force is transferred from one to the other. A hinge or bearing supports the axle, allowing rotation. It can amplify force; a small force applied to the periphery of the large wheel can move a larger load attached to the axle.
Greek philosophers such as Hero of Alexandria first identified the wheel and axle as one of the simple machines used to lift weights.[2] This is thought to have been in the form of the windlass which consists of a crank or pulley connected to a cylindrical barrel that provides mechanical advantage to wind up a rope and lift a load such as a bucket from the well.[3]
The wheel and axle can be viewed as a version of the lever, with a drive force applied tangentially to the perimeter of the wheel and a load force applied to the axle, respectively, that are balanced around the hinge which is the fulcrum. The mechanical advantage of the wheel and axle is the ratio of the distances from the fulcrum to the applied loads, or what is the same thing the ratio of the diameter of the wheel and axle.[4] A major application is in wheeled vehicles, in which the wheel and axle are used to reduce friction of the moving vehicle with the ground. Other examples of devices which use the wheel and axle are capstans, belt drives and gears.


History
The earliest well-dated depiction of a wheeled vehicle (a wagon—four wheels, two axles) is on the Bronocice pot, a ca. 3635–3370 BCE ceramic vase, excavated in a Funnelbeaker culture settlement in southern Poland.[5]
The oldest known example of a wooden wheel and its axle was found in 2002 at the Ljubljana Marshes some 20 km south of Ljubljana, the capital of Slovenia. According to radiocarbon dating, it is between 5,100 and 5,350 years old. The wheel was made of ash and oak and had a radius of 70 cm and the axle is 120 cm long and made of oak.[6]
Mechanical advantage
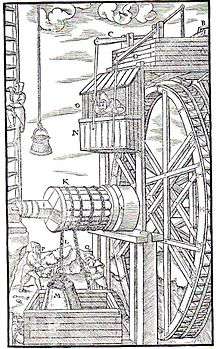
The simple machine called a wheel and axle refers to the assembly formed by two disks, or cylinders, of different diameters mounted so they rotate together around the same axis.The thin rod which needs to be turned is called the axle and the wider object fixed to the axle, on which we apply force is called the wheel. A tangential force applied to the periphery of the large disk can exert a larger force on a load attached to the axle, achieving mechanical advantage. When used as the wheel of a wheeled vehicle the smaller cylinder is the axle of the wheel, but when used in a windlass, winch, and other similar applications (see medieval mining lift to right) the smaller cylinder may be separate from the axle mounted in the bearings. It cannot be used separately.[7][8]
Assuming the wheel and axle does not dissipate or store energy, that is it has no friction or elasticity, the power input by the force applied to the wheel must equal the power output at the axle. As the wheel and axle system rotates around its bearings, points on the circumference, or edge, of the wheel move faster than points on the circumference, or edge, of the axle! Therefore, a force applied to the edge of the wheel must be less than the force applied to the edge of the axle, because power is the product of force and velocity.[9]
Let a and b be the distances from the center of the bearing to the edges of the wheel A and the axle B. If the input force FA is applied to the edge of the wheel A and the force FB at the edge of the axle B is the output, then the ratio of the velocities of points A and B is given by a/b, so the ratio of the output force to the input force, or mechanical advantage, is given by
The mechanical advantage of a simple machine like the wheel and axle is computed as the ratio of the resistance to the effort. The larger the ratio the greater the multiplication of force (torque) created or distance achieved. By varying the radii of the axle and/or wheel, any amount of mechanical advantage may be gained.[4] In this manner, the size of the wheel may be increased to an inconvenient extent. In this case a system or combination of wheels (often toothed, that is, gears) are used. As a wheel and axle is a type of lever, a system of wheels and axles is like a compound lever.[10]
Ideal mechanical advantage
The ideal mechanical advantage of a wheel and axle is calculated with the following formula:
Actual mechanical advantage
The actual mechanical advantage of a wheel and axle is calculated with the following formula:
where
- R = resistance force, i.e. the weight of the bucket in this example.
- Eactual = actual effort force, the force required to turn the wheel.
References
- ↑ Wheel and Axle, The World Book Encyclopedia, World Book Inc., 1998, pp. 280-281
- ↑ Usher, Abbott Payson (1988). A History of Mechanical Inventions. USA: Courier Dover Publications. p. 98. ISBN 048625593X.
- ↑ Elroy McKendree Avery, Elementary Physics, New York : Sheldon & Company, 1878.
- 1 2 Bowser, Edward Albert, 1890, An elementary treatise on analytic mechanics: with numerous examples. (Originally from the University of Michigan) D. Van Nostrand Company, pp. 190
- ↑ Anthony, David A. (2007). The horse, the wheel, and language: how Bronze-Age riders from the Eurasian steppes shaped the modern world. Princeton, N.J: Princeton University Press. p. 67. ISBN 0-691-05887-3.
- ↑ Aleksander Gasser (March 2003). "World's Oldest Wheel Found in Slovenia". Government Communication Office of the Republic of Slovenia. Retrieved 19 August 2010.
- ↑ Prater, Edward L. (1994), Basic Machines, Naval Education and Training Professional Development and Technology Center, NAVEDTRA 14037
- ↑ Bureau of Naval Personnel, 1971, Basic Machines and How They Work, Dover Publications.
- ↑ J. J. Uicker, G. R. Pennock, and J. E. Shigley, 2003, Theory of Machines and Mechanisms, Oxford University Press, New York.
- ↑ Baker, C.E. The Principles and Practice of Statics and Dynamics … for the Use of Schools and Private Students. London: John Weale, 59, High Holborn. 1851 pp. 26-29 read online or download full text
Additional Resources
Basic Machines and How They Work, United States. Bureau of Naval Personnel, Courier Dover Publications 1965, pp. 3–1 and following preview online