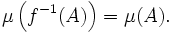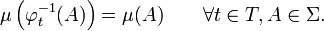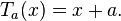Invariant measure

In mathematics, an invariant measure is a measure that is preserved by some function. Ergodic theory is the study of invariant measures in dynamical systems. The Krylov–Bogolyubov theorem proves the existence of invariant measures under certain conditions on the function and space under consideration.

Definition

Let (X, Σ) be a measurable space and let f be a measurable function from X to itself. A measure μ on (X, Σ) is said to be invariant under f if, for every measurable set A in Σ,

In terms of the push forward, this states that f_∗(μ) = μ.

The collection of measures (usually probability measures) on X that are invariant under f is sometimes denoted M_f(X). The collection of ergodic measures, E_f(X), is a subset of M_f(X). Moreover, any convex combination of two invariant measures is also invariant, so M_f(X) is a convex set; E_f(X) consists precisely of the extreme points of M_f(X).

In the case of a dynamical system (X, T, φ), where (X, Σ) is a measurable space as before, T is a monoid and φ : T × X → X is the flow map, a measure μ on (X, Σ) is said to be an invariant measure if it is an invariant measure for each map φ_t : X → X. Explicitly, μ is invariant if and only if

Put another way, μ is an invariant measure for a sequence of random variables (Z_t)_t≥0 (perhaps a Markov chain or the solution to a stochastic differential equation) if, whenever the initial condition Z₀ is distributed according to μ, so is Z_t for any later time t.

Examples

Squeeze mapping leaves hyperbolic angle invariant as it moves a hyperbolic sector (purple) to one of the same area. Blue and green rectangles also keep the same area

• Consider the real line R with its usual Borel σ-algebra; fix a ∈ R and consider the translation map T_a : R → R given by:

Then one-dimensional Lebesgue measure λ is an invariant measure for T_a.

• More generally, on n-dimensional Euclidean space Rⁿ with its usual Borel σ-algebra, n-dimensional Lebesgue measure λⁿ is an invariant measure for any isometry of Euclidean space, i.e. a map T : Rⁿ → Rⁿ that can be written as

for some n × n orthogonal matrix A ∈ O(n) and a vector b ∈ Rⁿ.

• The invariant measure in the first example is unique up to trivial renormalization with a constant factor. This does not have to be necessarily the case: Consider a set consisting of just two points 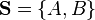and the identity map 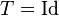which leaves each point fixed. Then any probability measure 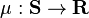is invariant. Note that S trivially has a decomposition into T-invariant components {A} and {B}.
• The measure of circular angles in degrees or radians is invariant under rotation. Similarly, the measure of hyperbolic angle is invariant under squeeze mapping.
• Area measure in the Euclidean plane is invariant under 2 × 2 real matrices with determinant 1, also known as the special linear group SL(2,R).
• Every locally compact group has a Haar measure that is invariant under the group action.

See also

• Quasi-invariant measure

References

• Invariant measures, John Von Neumann, AMS Bookstore, 1999, ISBN 978-0-8218-0912-9